A.0.8 Tensor
Beispiel-Aufgaben
Passive Transformation: 2.2.C
Aktive Transformation: 2.2.E
Videos
Aktive Transformation
Merkzettel: Aktiv = 1 Bezugssystem, 2 Tensoren, Pfeildrehung 2α
Es gibt:
das \(\left(x, y\right)\)-System
den Tensor \(\boldsymbol T\) und den Tensor \(\boldsymbol T'\)
den Winkel \(\alpha\), um den \(\boldsymbol T'\) relativ zu \(\boldsymbol T\) gedreht ist
\(\begin{bmatrix} T_{xx} & T_{xy} \\ \mathsf{sym} & T_{yy} \end{bmatrix}\): Die \(\left(x, y\right)\)-Komponenten des Tensors
\(\begin{bmatrix} T'_{xx} & T'_{xy} \\ \mathsf{sym} & T'_{yy} \end{bmatrix}\): Die \((x, y)\)-Komponenten des gedrehten Tensors
mit \(R_\alpha = \begin{bmatrix}c_\alpha & -s_\alpha \\s_\alpha & c_\alpha\end{bmatrix}\) und \(R_\alpha^{\mathsf T}\) als Transponierte von \(R_\alpha\).
Gleichung (1) ist äquivalent zu folgenden drei Aussagen:
mit den Abkürzungen:
Gegeben: Berechnung der gesuchten Komponenten mit (1): Berechnung der gesuchten Komponenten mit (1a) und (1b) und (1c): Ablesen der gesuchten Komponenten bei \(P\) und \(P'\): \(P\) hat die Koordinaten \((T'_{xx}, T'_{xy}) \stackrel{\small{0{,}01}}{\approx} (-3{,}94, 1{,}3)\). \(P'\) hat die Koordinaten \((T'_{yy}, -T'_{xy}) \stackrel{\small{0{,}01}}{\approx} (6{,}94, -1{,}3)\). Der dunkelgraue Pfeil mit den Komponenten \(( \tilde T, T_{xy} )\) aktiv gedreht mit Drehwinkel \(2\alpha\) zeigt auf den Punkt \(P.\) Denn (1a) und (1b) lassen sich auch schreiben als: Der dunkelgraue Pfeil aktiv gedreht mit Drehwinkel \(2\alpha\pm 180^\circ\) zeigt auf den Punkt \(P'\), denn (1c) und (1b) lassen sich auch schreiben als:Beispiel 1
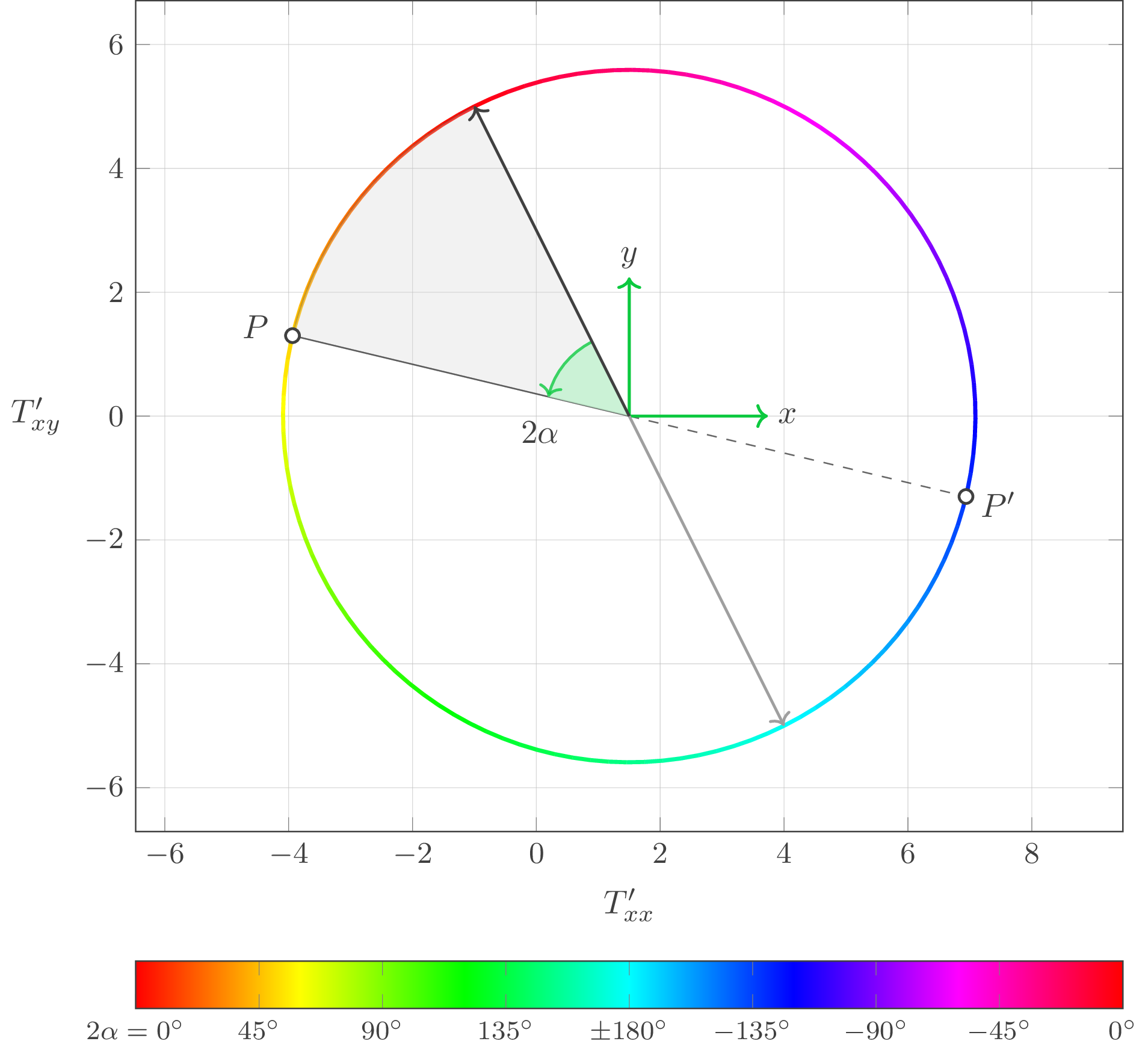
Zeigerdrehung
Beispiel 2
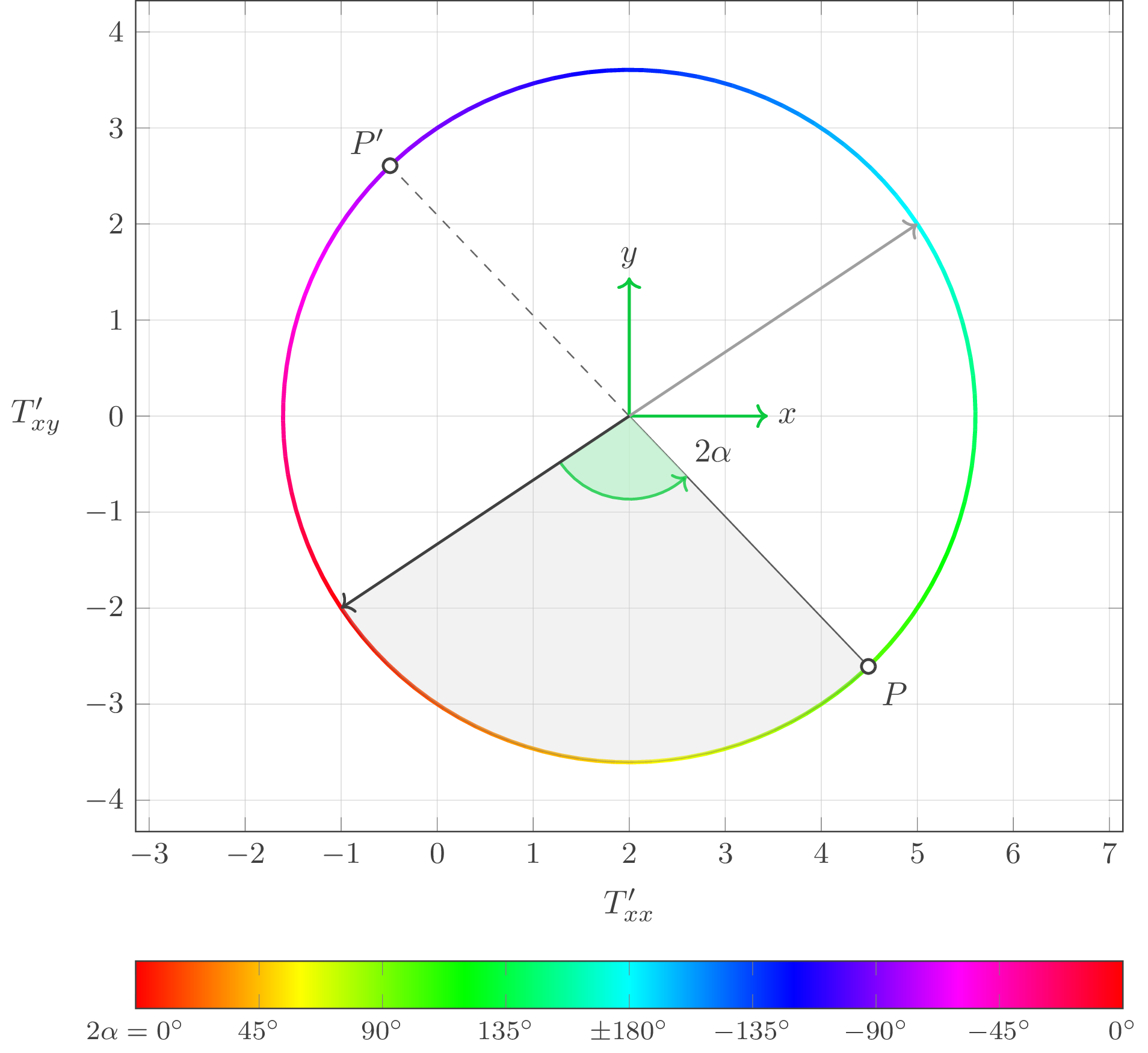
Beispiel 3

Passive Transformation
Merkzettel: Passiv = 2 Bezugssysteme, 1 Tensor, 1 Bezugssystem, Pfeildrehung -2φ
Es gibt:
das \(\left(x, y\right)\)-System und das \(\left(\bar x, \bar y\right)\)-System
den Winkel \(\varphi\), um den das \(\left(\bar x, \bar y\right)\)-System relativ zum \((x, y)\)-System gedreht ist
\(\begin{bmatrix} T_{xx} & T_{xy} \\ \mathsf{sym} & T_{yy} \end{bmatrix}\): Die \(\left(x, y\right)\)-Komponenten des Tensors
\(\begin{bmatrix} T_{\bar x \bar x} & T_{\bar x\bar y} \\ \mathsf{sym} & T_{\bar y\bar y} \end{bmatrix}\): Die \((\bar x, \bar y)\)-Komponenten des Tensors
mit \(R_\varphi = \begin{bmatrix}c_\varphi & s_\varphi \\-s_\varphi & c_\varphi\end{bmatrix}\) und \(R_\varphi^{\mathsf T}\) als Transponierte von \(R_\varphi\).
Gleichung (2) ist äquivalent zu folgenden drei Aussagen:
mit den Abkürzungen:
Gegeben: Berechnung der gesuchten Komponenten mit (2): Berechnung der gesuchten Komponenten mit (2a) und (2b) und (2c): Ablesen der gesuchten Komponenten bei \(P\) und \(P'\): \(P\) hat die Koordinaten \((T_{\bar x \bar x}, T_{\bar x \bar y}) \stackrel{\small{0{,}01}}{\approx} ( 1{,}9, -4{,}1)\). \(P'\) hat die Koordinaten \((T_{\bar y \bar y}, -T_{\bar x \bar y})\stackrel{\small{0{,}01}}{\approx} ( 4{,}1, 4{,}1)\). Der dunkelgraue Pfeil mit den Komponenten \(( \tilde T, T_{xy} )\) aktiv gedreht mit Drehwinkel \(-2\varphi\) zeigt auf den Punkt \(P.\) Denn (2a) und (2b) lassen sich auch schreiben als: Der dunkelgraue Pfeil aktiv gedreht mit Drehwinkel \(-2\varphi\pm 180^\circ\) zeigt auf den Punkt \(P'\), denn (2c) und (2b) lassen sich auch schreiben als:Beispiel 1

Zeigerdrehung
Beispiel 2

Beispiel 3
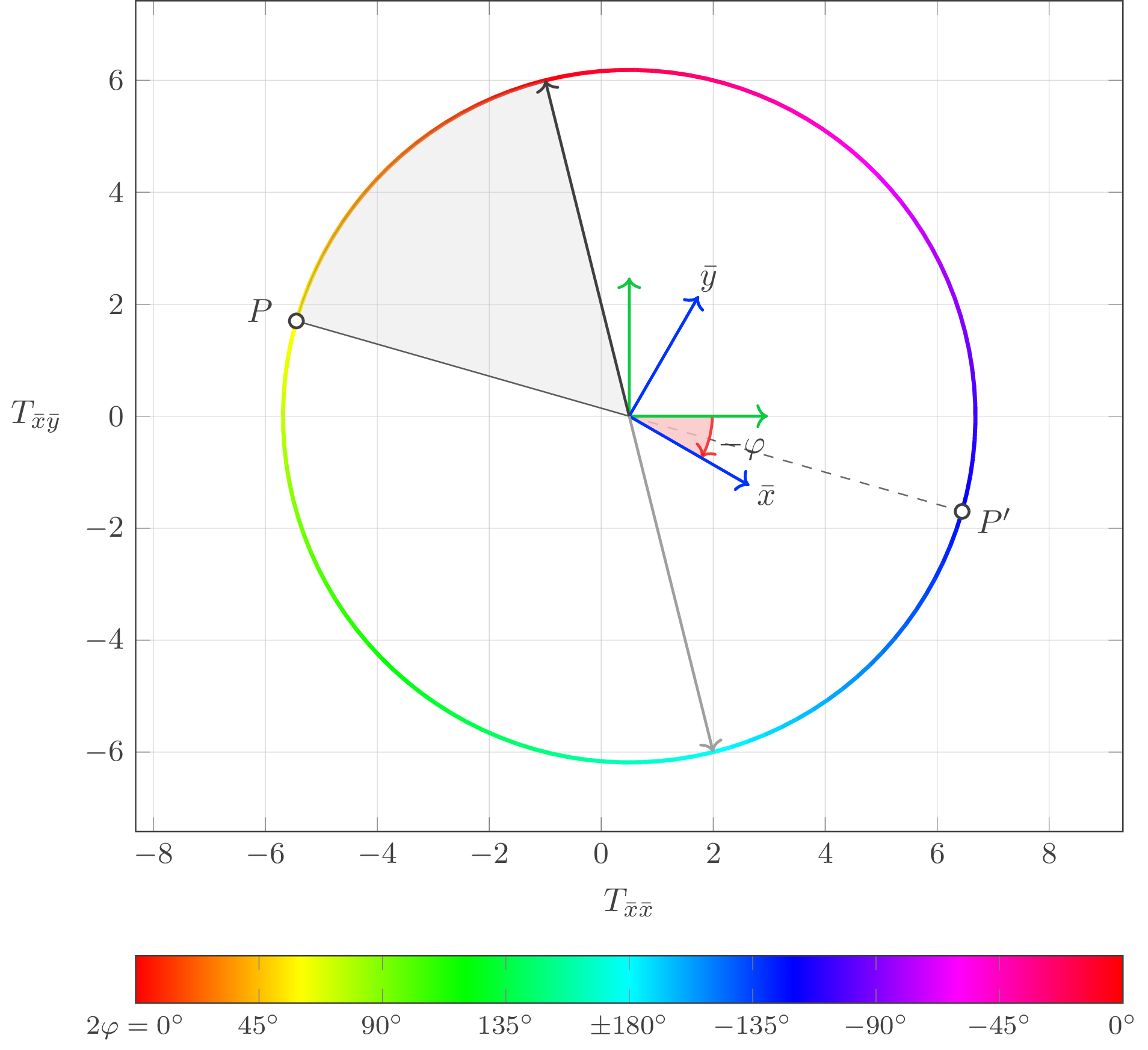
Passive vs. Aktive Transformation
Gleiche Komponenten, aber andere Bedeutung
Für \(\alpha=-\varphi\) bzw. gleichbedeutend \(\varphi = - \alpha\) gilt:
Eine passive Transformation mit \(\varphi=30^\circ\) führt auf dieselben transformierten Komponenten wie eine aktive Transformation (desselben Vektors) mit \(\alpha = -30^\circ\).
Das Bezugssystem um 30 Grad entgegen dem Uhrzeigersinn zu drehen führt auf dieselben transformierten Komponenten wie den Tensor um 30 Grad im Uhrzeigersinn zu drehen.
Die transformierten Komponenten sind hierbei zahlenmäßig gleich - haben aber verschiedene Bedeutungen.
Web-App
Für Passive Transformation: Die gegebenen Komponenten \((T_{xx}, T_{xy}, T_{yy})\) eintragen als einheitenlose Größen. Falls z.B. ein Tensor untersucht werden soll mit den Komponenten \(\left(2\,\mathrm{Pa}, 1\,\mathrm{Pa}, \tfrac 3 2 \,\mathrm{Pa}\right)\): Dann würde man oben eintragen: \((T_{xx}, T_{xy}, T_{yy}) = (2, 1, 1.5).\) Den Winkel \(\varphi\) einstellen. Die gesuchten Komponenten \((T_{\bar x \bar x}, T_{\bar x\bar y})\) im Diagramm ablesen. Auch \(T_{\bar y \bar y}\) lässt sich ablesen, und zwar beim Punkt gegenüber auf dem Mohrschen Kreis. Dort ist der Winkel um 90 Grad erhöht oder verringert gegenüber dem gegebenen Winkel. Es gilt: Beispiel: 180-Grad-Periodizität: Die Passive Transformation ist definiert für jeden beliebigen Winkel \(\varphi\). Denn es gilt für jedes beliebige \(n\in\mathbb{N}:\) Beispiel:Anleitung
Nachfolgend ein Programm, dass Sie ausführen können: Auf dem PC z.B. mit Anaconda. Im Browser (online) in drei Schritten: Copy: Source Code in die Zwischenablage kopieren. Paste: Source Code als Python-Notebook einfügen z.B. auf: JupyterLab oder Play: Ausführen. Statt SymPy lieber anderes CAS (Computeralgebrasystem) verwenden? Eine Auswahl verschiedener CAS gibt es hier.SymPy
from sympy import pi, Matrix, cos, sin, pprint, sqrt, atan
deg = pi/180
# Rounding:
def iso_round(x, pv):
try:
x = float(x)
tmp = round(x, pv)
except TypeError:
from functools import partial
func = partial(round, ndigits = pv)
tmp = x.applyfunc(func)
return tmp
Txx, Txy, Tyy = 2, 1, 1.5
# Output precision:
prec = 2
pprint("\nComponents (Txx, Txy, Tyy):")
T = (Txx, Txy, Tyy)
pprint(T)
pprint("\nComponent-Matrix:")
T = Matrix([[Txx, Txy], [Txy, Tyy]])
pprint(T)
pprint("\nAngle / deg:")
angle = 40 * deg
tmp = angle / deg
tmp = iso_round(tmp,prec)
pprint(tmp)
c, s = cos(angle), sin(angle)
R = Matrix([[c, s],[-s, c]])
passive=False
passive=True
if passive:
pprint("\nComponents (Tx̄x̄, Tx̄ȳ, Tȳȳ):")
tmp = R*T*R.transpose()
else:
pprint("\nComponents (T'xx, T'xy, T'yy):")
tmp = R.transpose()*T*R
T = iso_round(tmp,prec)
tmp = (T[0,0], T[0,1], T[1,1])
pprint(tmp)
pprint("\nComponent-Matrix:")
pprint(T)
# # Or use Calculator:
# if mode == "active":
# p*=-1
# # Define Shortcuts:
# c = cos(2*p)
# s = sin(2*p)
# Tb = (Txx + Tyy) / 2
# Tt = (Txx - Tyy) / 2
# # Use Shortcuts:
# T11 = Tb + Tt*c + Txy*s
# T12 = -Tt*s + Txy*c
# T22 = Tb - Tt*c - Txy*s
# tmp = Matrix([[T11, T12], [T12, T22]])
# tmp = iso_round(tmp, digits)
# pprint(tmp)
if passive:
pprint("\nTm:")
Tm = (Txx + Tyy)/2
tmp = Tm
tmp = iso_round(tmp,prec)
pprint(tmp)
pprint("\nr:")
r = ( (Txx - Tyy) / 2 )**2 + Txy**2
tmp = sqrt(r)
tmp = iso_round(tmp,prec)
pprint(tmp)
pprint("\nmax Tx̄x̄:")
tmp = Tm + r
tmp = iso_round(tmp,prec)
pprint(tmp)
pprint("\nmin Tx̄x̄:")
minT = Tm - r
tmp = minT
tmp = iso_round(tmp,prec)
pprint(tmp)
pprint("\nφ₁ / deg:")
p1 = atan(Txy/(Txx - minT))
tmp = p1
tmp /= deg
tmp = iso_round(tmp,prec)
pprint(tmp)
pprint("\nmax Tx̄ȳ:")
tmp = r
tmp = iso_round(tmp,prec)
pprint(tmp)
pprint("\n(φ₁ - 45°) / deg:")
tmp = p1 / deg - 45
tmp = iso_round(tmp,prec)
pprint(tmp)
Components (Txx, Txy, Tyy):
(2, 1, 1.5)
Component-Matrix:
⎡2 1 ⎤
⎢ ⎥
⎣1 1.5⎦
Angle / deg:
40.0
Components (Tx̄x̄, Tx̄ȳ, Tȳȳ):
(2.78, -0.07, 0.72)
Component-Matrix:
⎡2.78 -0.07⎤
⎢ ⎥
⎣-0.07 0.72 ⎦
Tm:
1.75
r:
1.03
max Tx̄x̄:
2.81
min Tx̄x̄:
0.69
φ₁ / deg:
37.3
max Tx̄ȳ:
1.06
(φ₁ - 45°) / deg:
-7.7
Andere Ebenen und 3D
Entsprechende Formeln lassen sich auch für die anderen Ebenen aufstellen. Hierbei wird zyklisch vertauscht.
Ebene |
\(\varphi\) zählt pos. um |
|---|---|
\((x,y)\) |
\(z\) |
\((y,z)\) |
\(x\) |
\((z,x)\) |
\(y\) |
Beispiel \((y,z)\)-Ebene
Passive und aktive Transformation:
Für 3D siehe 3D Elementar-Drehungen.
Weitere Eigenschaften der Passiven Transformation siehe hier.
Tensor-Komponente
Eine Tensor-Komponente ist der Anteil eines symmetrischen Tensors in einer bestimmten „Richtung“.
Diese „Richtung“ wird definiert mit zwei Einheitsvektoren (entlang dieser „Richtung“).
Um eine Tensor-Komponenten zu berechnen, braucht man:
ein Bezugssystem, z.B. das \((x,y,z)\)-System.
die Komponenten des Tensors (bezüglich Bezugssystem): \(T_{xx}, T_{xy}, T_{xz}, T_{yy}, T_{yz}, T_{zz}\).
die Komponenten der Einheitsvektoren (bezüglich Bezugssystem).
\(xx\)-Komponente
Sei die „Richtung“ die \(xx\)-„Richtung“. Dann gilt für die Tensor-Komponente \(T_{xx}\) in dieser „Richtung“:
2D
In 2D z.B. in der \((x,y)\)-Ebene:
\(xy\)-Komponente
Sei die „Richtung“ die \(xy\)-„Richtung“. Dann gilt für die Tensor-Komponente \(T_{xy}\) in dieser „Richtung“:
2D
In 2D z.B. in der \((x,y)\)-Ebene:
\(\bar x\bar x\)-Komponente
Sei die „Richtung“ die \(\bar x\bar x\)-„Richtung“. Weil \(\bar x\) um den Winkel \(\varphi\) relativ zu \(x\) gedreht ist, hat ein Einheitsvektor in \(\bar x\)-Richtung die \((x,y,z)\)-Komponenten \((c_\varphi, s_\varphi, 0)\). Und für die Tensor-Komponente \(T_{\bar x \bar x}\) in dieser „Richtung“ gilt:
2D
In 2D z.B. in der \((x,y)\)-Ebene:
\(\bar x\bar y\)-Komponente
Sei die „Richtung“ die \(\bar x\bar y\)-„Richtung“. Weil \(\bar x\) um den Winkel \(\varphi\) relativ zu \(x\) gedreht ist, hat ein Einheitsvektor in \(\bar x\)-Richtung die \((x,y,z)\)-Komponenten \((c_\varphi, s_\varphi, 0)\). Und weil \(\bar y\) um den Winkel \(\varphi\) relativ zu \(y\) gedreht ist, hat ein Einheitsvektor in \(\bar y\)-Richtung die \((x,y,z)\)-Komponenten \((-s_\varphi, c_\varphi, 0)\). Und für die Tensor-Komponente \(T_{\bar x \bar y}\) in dieser „Richtung“ gilt:
2D
In 2D z.B. in der \((x,y)\)-Ebene:
Dieselben Formeln werden in der Passiven Transformation verwendet.